Time: 3 pm Eastern/noon Pacific
Title: The geometry of number theory, through Mobius transformations
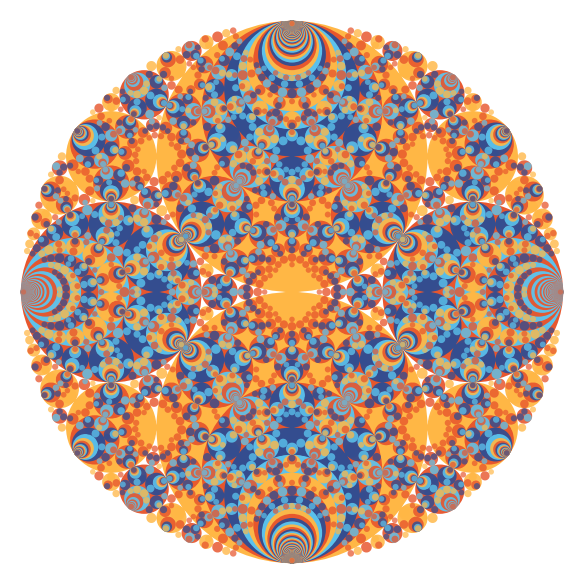
Abstract: Mobius transformations beautifully illustrate the geometry of complex numbers. My own interest arose when playing with some questions from number theory. I’ll show you some of the hidden geometry of number theory through Schmidt arrangements, which are fractal circle packings built of Mobius transformations. Along the way, I’ll share my own story of computer-driven mathematical experimentation and illustration.
Bio: Katherine Stange is a number theorist, cyclist and mom at the University of Colorado, Boulder. Mathematically speaking, she likes to dig around in the mud and get messy, with examples and computer data. She enjoys number theory’s diverse tools in the face of elementary problems, loves to explore mathematics visually and computationally, and is attracted to problems involving geometry. She also enjoys algorithms and works on post-quantum cryptography. She did her Bachelor of Mathematics at the University of Waterloo, and her PhD at Brown University.